Maths
Intent
Mathematics is at the core of our curriculum and it is through our teaching in this subject that pupils learn crucial life skills, including resilience and problem-solving. Through a wide range of approaches, it is our intent that all children become fluent in each area of the maths curriculum so they can proceed to engage in higher-level reasoning and problem-solving skills, both within the maths lesson and across the wider curriculum. Our aim is to provide opportunities for all of our learners to lead, teach, support and mentor each other in order to further embed and deepen their mathematical thinking and to develop their confidence as mathematicians.
Implementation
The Early Years
Early maths skills are reinforced through practical activities, often outside, and using a wide range of physical resources, often linked to role-play and developed through enhanced provision. Challenges posed encourage problem-solving and application of their learning; this is embedded further through weekly Forest School sessions.
Building on a solid foundation
To ensure consistency and a clear progression of skills, we follow the White Rose Maths small steps scheme. Children complete pre-assessments before each unit of work to guide their individual learning journeys. In all sessions, variation is paramount and provided through: different starting points, a range of challenge, the support of practical resources and groupings. Children may work with a teacher for guided maths, independently to access activities of an appropriate level of challenge, in groups to support each other or as part of a whole class to draw their learning together.
To supplement the spiral, core provision (White Rose Maths), a wide range of additional resources are used to broaden and deepen the children's learning. Re-cap activities, investigations and problem-solving that requires skills beyond the current unit of work are all part of the learning journey, captured in workbooks and exercise books. New knowledge, concepts, strategies, language and stem sentences (sentences that are always true) are mapped-out with the children on class learning walls, which support the teaching and learning throughout a unit. As the children become more independent in their learning, Maths Journals are used to capture the content of these learning walls (whether through photographs or individual note-taking), supporting autonomous learning by acting as a reminder when a topic is revisited.
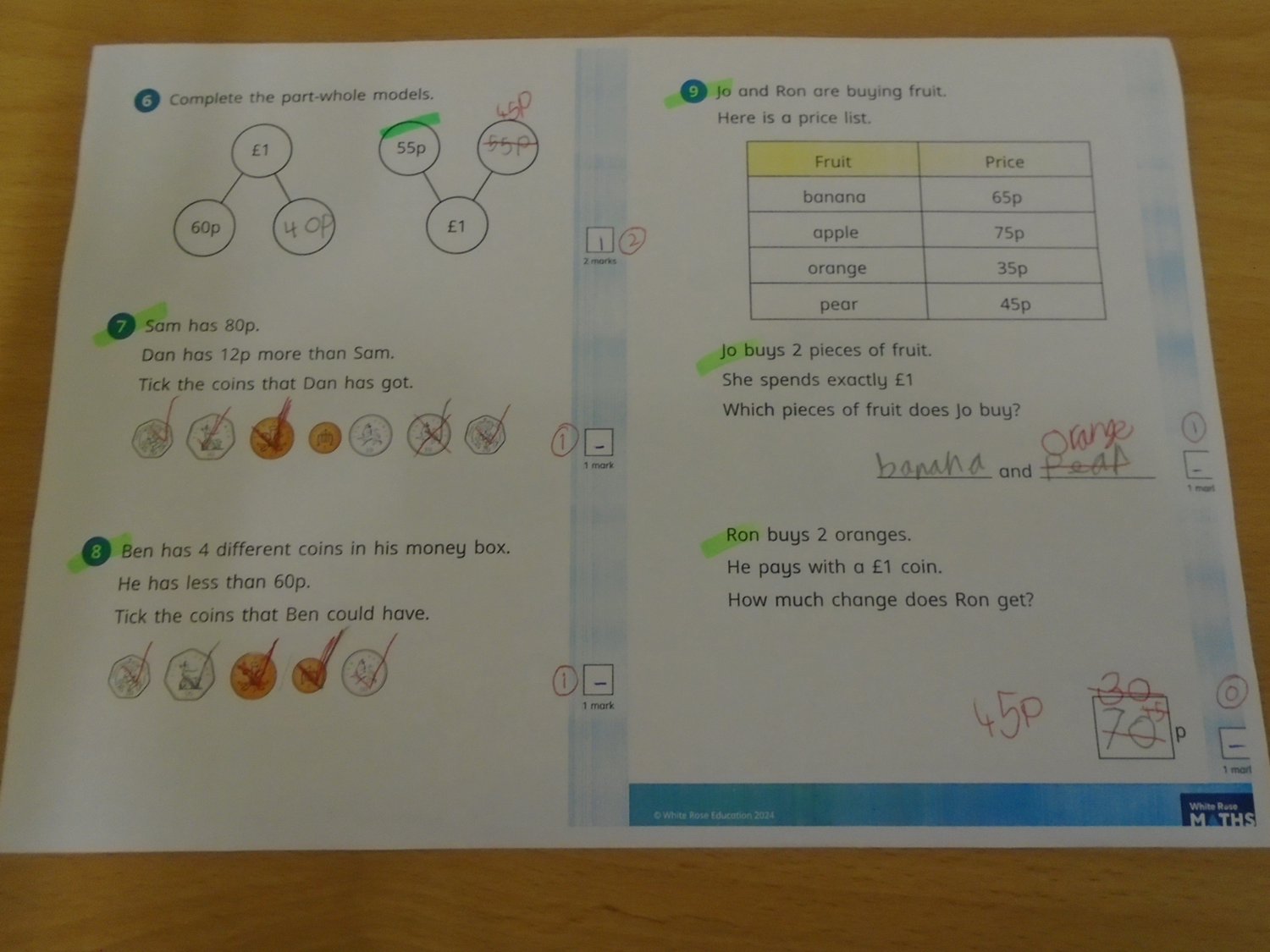
When appropriate, children are taught to mark their own work as they progress through the session (in red) in order to identify misconceptions immediately or move them onto a higher level of challenge. Mistakes are either self-corrected, where the child understands their error, or worked through with a partner or adult as needed. If required, additional fluency practice is provided; for others additional 'Chilli challenges' are offered to stretch and deepen understanding.
Concrete: In this stage, children are first introduced to an idea or skill by acting it out with real objects. For example, in division this might be done by separating balls into colour groups.
Pictorial: When a child has sufficiently understood the
Abstract: This is the symbolic stage, where the child is able to represent problems using mathematical notations e.g. 10÷5=2. The children will only progress to the abstract stage when they have enough context to understand what they mean, as this is the 'final' and most challenging of the three stages.
SEND: supporting understanding and independence for all
Our brains work in different ways and therefore require different approaches to making sense of maths. In addition to the approaches to variation referenced above, different supported scaffolding approaches may be used at any stage, along with overlearning tasks or more support from an adult. These will be used as necessary for the individual to make sense of the learning and become independent.
Impact
As children move through their learning journeys, they become increasingly fluent in the skills and are able to articulate their learning. This progress is reflected in their workbooks and captured in their post assessments: pre-assessments from the beginning of the topic are returned to, to be corrected and /or completed. The child and teacher are then able to celebrate progress, plan further support and share achievements by taking these home.
How do I help my Child?
It can be tricky to know how to help your child without confusing them further; maths today may not be what you remember from your own school days. To help we have attached our progression of mental and written methods document as a guide to how these are developed at Langrish, from the Early Years, through the Infants and beyond. Please use this as a manual when helping your child with their home learning. We have also added two documents with links - one a page of useful websites to further the children's learning, the other with a list of videos to show you how we introduce different skills using the concrete-pictorial-abstract approach. These are listed by objective and are age-appropriate. If you have any further questions, don't be afraid to come and ask!
Here are some useful websites to help support your child's maths learning at home.






